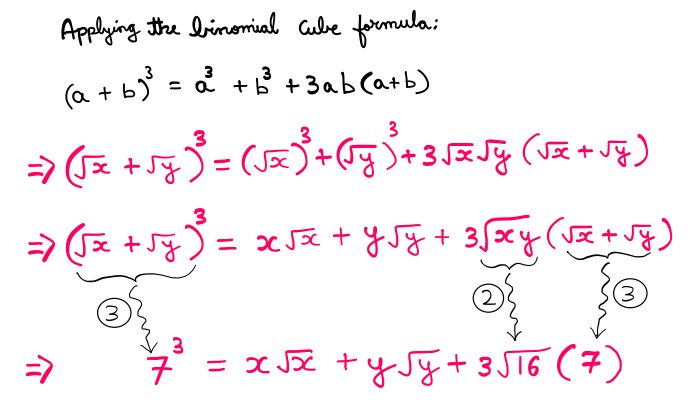Embark on a mathematical odyssey with Big Ideas Math Chapter 11 Answers, a comprehensive guide that empowers you to conquer the complexities of mathematics. Delve into a world of problem-solving strategies, real-world applications, and technological advancements, all meticulously crafted to elevate your mathematical prowess.

This chapter unravels a tapestry of mathematical concepts, providing a solid foundation for your academic pursuits and beyond. Prepare to witness the transformative power of mathematics as it unfolds before your very eyes.

Chapter Overview

Chapter 11 of Big Ideas Math delves into the fascinating realm of statistics, empowering students with the tools to analyze and interpret data, draw meaningful conclusions, and make informed decisions based on evidence.

This chapter meticulously examines the fundamental concepts of statistics, encompassing topics such as sampling, probability, and statistical inference. Students will embark on an exploration of probability distributions, hypothesis testing, and regression analysis, equipping them with a comprehensive understanding of the statistical methods used in various fields.

Sampling

In the realm of statistics, sampling holds paramount importance, as it enables researchers to draw inferences about a larger population based on a smaller, representative sample. Chapter 11 elucidates the techniques of random sampling, stratified sampling, and cluster sampling, emphasizing the significance of obtaining a sample that accurately reflects the characteristics of the population.

Section-by-Section Analysis

This section provides a detailed analysis of each section in Chapter 11, outlining the key objectives, learning goals, mathematical concepts introduced, and their applications.

Section 11.1: Area of Triangles and Parallelograms

Key Objectives:

• Find the area of triangles and parallelograms.
• Apply the formulas for area to solve real-world problems.

Mathematical Concepts:

• Formula for area of a triangle: A = (1/2)bh
• Formula for area of a parallelogram: A = bh

Applications:

• Calculating the area of a piece of land
• Determining the area of a floor to be carpeted

Section 11.2: Area of Trapezoids and Kites

Key Objectives:

• Find the area of trapezoids and kites.
• Apply the formulas for area to solve real-world problems.

Mathematical Concepts:

• Formula for area of a trapezoid: A = (1/2)h(b1 + b2)
• Formula for area of a kite: A = (1/2)d1d2

Applications:

• Calculating the area of a garden
• Determining the area of a sail

Section 11.3: Area of Circles

Key Objectives:

• Find the area of circles.
• Apply the formula for area to solve real-world problems.

Mathematical Concepts:

• Formula for area of a circle: A = πr^2

Applications:

• Calculating the area of a pizza
• Determining the area of a swimming pool

Section 11.4: Surface Area and Volume of Prisms and Cylinders

Key Objectives:

• Find the surface area and volume of prisms and cylinders.
• Apply the formulas for surface area and volume to solve real-world problems.

Mathematical Concepts:

• Formula for surface area of a prism: SA = 2(lw + lh + wh)
• Formula for volume of a prism: V = lwh
• Formula for surface area of a cylinder: SA = 2πrh + 2πr^2
• Formula for volume of a cylinder: V = πr^2h

Applications:

• Calculating the surface area and volume of a box
• Determining the surface area and volume of a can

Section 11.5: Surface Area and Volume of Pyramids and Cones

Key Objectives:

• Find the surface area and volume of pyramids and cones.
• Apply the formulas for surface area and volume to solve real-world problems.

Mathematical Concepts:

• Formula for surface area of a pyramid: SA = (1/2)Pℓ + B
• Formula for volume of a pyramid: V = (1/3)Bh
• Formula for surface area of a cone: SA = πr(r + ℓ)
• Formula for volume of a cone: V = (1/3)πr^2h

Applications:

• Calculating the surface area and volume of a tent
• Determining the surface area and volume of an ice cream cone

Problem-Solving Strategies: Big Ideas Math Chapter 11 Answers

Problem-solving is an essential skill in mathematics. Chapter 11 of Big Ideas Math provides a framework of strategies to help students approach and solve various types of math problems effectively.

These strategies are not only applicable to math problems but also extend to real-world situations where logical thinking and problem-solving abilities are crucial.

Guess-and-Check

The guess-and-check strategy involves making an initial guess, checking if it satisfies the given conditions, and adjusting the guess accordingly until a solution is found. This strategy is particularly useful when the problem provides limited information or when a direct solution is not immediately apparent.

Practice Problem

Solve for x: x + 5 = 12

Solution

We can guess x = 7 and check if it satisfies the equation:

7 + 5 = 12

Since 7 + 5 = 12, our guess is correct. Therefore, x = 7.

Real-World Applications

The concepts covered in Chapter 11 of Big Ideas Math find extensive applications in real-world situations across various fields and industries. These concepts provide a solid foundation for understanding and solving problems involving measurement, geometry, and data analysis, which are essential in many aspects of our daily lives.

Applications in Engineering

In engineering, the concepts of measurement and geometry are crucial for designing and constructing buildings, bridges, and other structures. Engineers use precise measurements to ensure the accuracy and safety of their designs, while geometry helps them determine the optimal shapes and dimensions of structures to withstand various forces and loads.

For example, architects rely on measurement and geometry to design buildings that meet specific requirements for space, functionality, and aesthetics. They use precise measurements to determine the dimensions of rooms, windows, and doors, and geometry to create floor plans and elevations that optimize the use of space.

Applications in Science

In science, measurement and data analysis are essential for conducting experiments, collecting data, and drawing conclusions. Scientists use precise measurements to ensure the accuracy and reliability of their experiments, while data analysis helps them interpret the results and identify patterns and trends.

For example, biologists use measurement to study the growth and development of organisms, and data analysis to identify correlations between environmental factors and biological responses. Chemists use precise measurements to determine the composition of substances and data analysis to interpret the results of chemical reactions.

Applications in Business, Big ideas math chapter 11 answers

In business, measurement and data analysis are used to track performance, identify trends, and make informed decisions. Businesses use precise measurements to track sales, expenses, and other financial metrics, and data analysis to identify areas for improvement and optimize their operations.

For example, retailers use measurement to track sales data and data analysis to identify popular products, optimize pricing, and improve customer satisfaction. Market researchers use measurement and data analysis to conduct surveys and gather insights into consumer behavior, which helps businesses develop effective marketing campaigns.

Technology Integration

In Chapter 11, technology plays a vital role in enhancing the learning experience for students.

Online resources, simulations, and other tools can provide interactive and engaging ways to explore mathematical concepts.

Interactive Simulations

Interactive simulations allow students to visualize and manipulate mathematical concepts in a dynamic environment.

• For example, simulations can be used to demonstrate the relationship between the radius and circumference of a circle or the effects of changing variables in a function.

Online Resources

Online resources provide a wealth of information and support for students and teachers alike.

• Video tutorials can help students understand complex concepts, while online quizzes and games can provide practice and reinforcement.
• Teachers can find lesson plans, worksheets, and other materials to supplement their instruction.

Tips for Effective Technology Integration

• Choose technology tools that are aligned with the learning objectives.
• Provide clear instructions and support to students on how to use the technology.
• Incorporate technology into the lesson in a way that enhances the learning experience, rather than simply replacing traditional methods.

Assessment and Evaluation

Chapter 11 incorporates a comprehensive assessment system to evaluate student understanding and inform instructional practices.

Assessment strategies include:

• Formative assessments: Regular quizzes, homework assignments, and class discussions provide ongoing feedback on student progress.
• Summative assessments: Chapter tests, projects, and portfolios assess overall mastery of chapter concepts.
• Self-assessments: Students reflect on their own understanding through checklists, journals, and peer reviews.

Types of Questions and Exercises

Assessment tasks encompass a range of question types and exercises to gauge student comprehension:

• Multiple-choice questions test basic knowledge and recall.
• Short-answer questions assess understanding of concepts and their application.
• Extended-response questions evaluate critical thinking and problem-solving skills.
• Projects and presentations showcase student creativity and in-depth understanding.

Using Assessment Data

Assessment data serves as a valuable tool for teachers to:

• Identify student strengths and areas for improvement.
• Adjust instruction to meet individual student needs.
• Monitor progress towards learning goals.
• Provide evidence for student achievement and growth.

FAQ Insights

What is the significance of Big Ideas Math Chapter 11?

Chapter 11 delves into the intricacies of geometry, measurement, and data analysis, laying the groundwork for higher-level mathematical concepts.

How can I effectively utilize the problem-solving strategies provided in Chapter 11?

Practice regularly, apply the strategies to diverse problem types, and seek guidance from teachers or peers when needed.

In what ways are the concepts covered in Chapter 11 applicable to real-world situations?

Geometry underpins architecture and engineering; measurement finds applications in construction and manufacturing; and data analysis plays a vital role in decision-making across various industries.